Plotting Sheets

Plotting sheets are "charts" designed primarily for open ocean navigation, where shorelines, visual aids to navigation and water depths are of no importance to navigation. Plotting sheets are also used for plotting positions and Lines-of-Position obtained by methods of Celestial Navigation.

Commercially available plotting sheets contain a Mercator grid for a specific Latitude. This grid provides the correct ratio between the angular distances in Latitude and Longitude similar to the nautical charts used for navigation in the corresponding Latitude. In this way the same feature concerning measuring distances by evaluating the angular latitude distance is valid for these plotting sheets.

Sheets with a prepared Mercator Grid according to the mid-latitude of the chart are available from the Book with Mercator Plotting Sheets for a Latitude range from 0° to 69°. The plotting sheet is valid only for this specific value of Latitude but can be used on both hemispheres, North or South. The Meridians are initially not labelled, so each latitude-specific plotting sheet can be used for any Longitude. The Meridians - and also the remaining Latitudes - will be labelled such that the required plotting area (e.g. from initial to final plotting position) is available on the sheet.

Constructing Small-Area Plotting Sheets

A plotting sheet with a Mercator scale for a specific Latitude Lat not covering more than a couple of degrees of Latitude, can be simply made from a blank sheet of paper. There are two ways to do this depending on how the scale of the chart is fixed: by Latitude or by Longitude.

Plotting Sheet with selected latitude scale

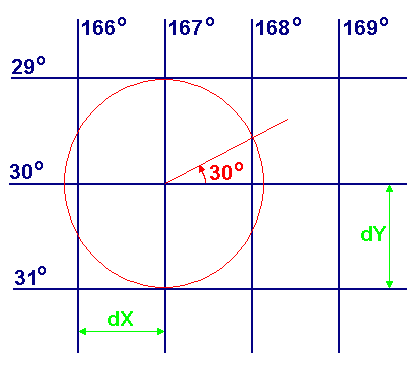
Draw a set of Parallels of equal Latitude as horizontal lines, spaced by a vertical distance of dY centimetres. Draw the primary Meridian as a vertical line intersecting the central Parallel at the center of the chart. Draw a circle at this center with a radius of dY. Label the central Parallel with the value of the corresponding Latitude Lat, (S 30° in the example below) and draw an inclined construction line originating from the center of the chart and inclined against the central Parallel by the latitude angle. Draw the next Meridian through the intersection point of the inclined construction line with the central circle. Complete the set of vertical meridian lines, spaced by a distance dX = dY * cos(Lat) centimetres as required for a Mercator Grid. Scale the chart by defining the size of dY: if dY represents one degree of Latitude then dX represents one degree of Longitude; if dY represents one minute of Latitude then dX represents one minute of Longitude. Label all Meridians and all Parallels of equal Latitude. Graduate the left and/or right Meridian into convenient units to enable distance measurement from the chart.

Move, and keep the cursor over the chart to see an animation of the construction principle

Plotting Sheet with selected longitude scale

Draw a set of lines of equal Longitude as vertical lines, spaced by an horizontal distance of dX centimetres. Draw a central horizontal line representing the Parallel of the mid-latitude of the plotting area. Label the central Parallel with the value of Latitude Lat, (S 30° in the example below) and draw an inclined construction line originating from the center of the chart and inclined against the central Parallel by the latitude angle. Draw a central circle through the point where the inclined construction line intersects with the next meridian line. Draw the upper and lower horizontal Parallels at the points where the central circle intersects with the central Meridian. Through this construction, the Parallels are spaced by a distance of dY = dX / cos(Lat) centimetres as required for a Mercator Grid. Scale the chart by defining dX: if dX represents one degree of Latitude then dY represents one degree of Longitude; if dX represents one minute of Latitude then dY represents one minute of Longitude. Label all the Meridians and all the lines of equal Latitude. Graduate the left or right Meridian line into convenient units to enable distance measurement from the chart.

Move, and keep the cursor over the chart to see an animation of the construction principle

Remarks

The above procedures differ only in the way the initial scale of the chart is defined. The first procedure has a predefined vertical spacing of the Parallels, whereas the second procedure allows for a predefined horizontal spacing of the Meridians.

The plotting sheet can be used as a usual nautical chart, for plotting course lines, lines of position, positions of the vessel etc. As in all Mercator charts, distances are obtained from the left- or right-hand scale of the plotting sheet. If the plotting sheet is constructed with selected latitude scale and dY is defined as one minute of Latitude per centimetre, distances can be simply taken from the sheet by using a ruler with a centimetre scale.

Notice: plotting sheets constructed with the above described techniques, use a constant ratio of Latitude spacing to Longitude spacing over the entire sheet. For this reason, they must be limited to a couple of degrees of Latitude, otherwise they will be unprecise.
The construction of large-scale Mercator grids is slightly more complicated and is described in the next section.

Constructing Large-Scale Plotting Sheets

In order to construct a plotting sheet, which is also valid for a large range of Latitudes, the basic characteristics of the Mercator grid must be understood:

• Meridians are straight lines running North-South and are spaced a fixed distance dX from each other.
  
• Parallels are also straight lines running East-West (perpendicular to the Meridians) and are spaced a distance dY from the next one. The value for dY depends on the value of the current Latitude Lat: dY = dX / cos(Lat). At the Equator, the Parallels are spaced at the same distance as the Meridians (dY = dX). The closer to the Poles, the larger the spacing of the Parallels (dY > dX).

Constructing the appropriate Mercator grid valid also for a large range of Latitudes may then be done by repeating the above procedure of constructing a plotting sheet with selected longitude scale for the different Latitude values available in the large-scale chart.

The Universal Plotting Sheet

The elaboration of a celestial fix according to the method of St. Hilaire does not necessarily require a Mercator style plotting sheet. I am indebted to Tony for sending me the following method, he found in an old marine textbook.

Instead of preparing a Mercator plotting sheet for doing the graphical elaboration of the Lines-of-Position and a fix, the "Universal Plotting Sheet" starts with a blank sheet of paper and an arbitrary distance scale, e.g. one centimetre per Nautical Mile. This scale is used over the entire sheet for both main directions: North-South and East-West. Notice that a Mercator Sheet would have different scales for these directions.

Near the center of the plotting sheet, the Assumed Position (AP) is chosen and the local Circle of Latitude and local Meridian is drawn as X and Y axis. The graphical elaboration of the celestial fix is performed the same way as on a Mercator Sheet: draw the Azimuth lines from the Assumed Position, draw the Lines-of-Position at the correct Altitude offsets and if required, shift the first LoP over the travelled distance between the two observations. This finally yields a fix with the current position (at the moment of the second observation).
Since this method does not use a Mercator Sheet, the coordinates of the graphically obtained position fix cannot be directly read from the plotting sheet's axis. Instead the offset of the fix to the Assumed Position must be evaluated. For the Latitude, the North-South distance between the fix and the Assumed Position can be directly translated into a difference in Latitude using the pre-defined chart scale:

  Latitude_Difference_in_Minutes = NS_Distance_in_Nautical_Miles

For the Longitude however, the correct ratio between Latitude and Longitude scales must be taken into account. Numerically, the ratio is equal to the cosine of the local Latitude (LatAP):

  Longitude_Difference_in_Minutes = EW_Distance_in_Nautical_Miles / cos(LatAP)

At higher Latitudes, the Meridians are "closer" than they are at the Equator, so at higher Latitudes, there are more "Minutes of Longitude per Mile" than there are at low Latitudes. Graphically, the correct Longitude difference can be obtained from the East-West distance with the following simple trigonometric construction:

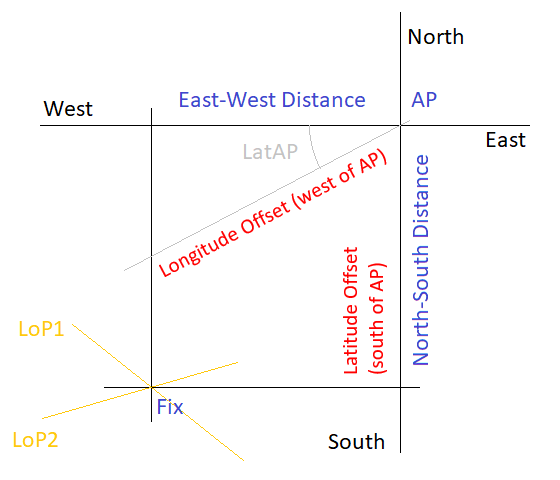
The scale of the plotting sheet is used to translate the Latitude and Longitude offsets (shown in red in the sketch above) to Minutes-of-Arc with one Nautical Mile corresponding to one Minute-of-Arc.